A canonical analysis determines a number of equations, each of the scalar form (sticking to my notation):
Thus, canonical analysis solves the operational problem: how does one best define the independent situations in which situational expectations, perceptions, dispositions, and distances (interests and capabilities) are maximally linked. Moreover, canonical analysis also solves the problem of how to measure expectations and perception. These turn out to be the canonical coefficients, and are thus measured in terms of the empirical relationship between behavioral dispositions and distance vectors.3
These 71 analyses provide 197 canonical equations (situations) linking expectations, behavioral dispositions, perceptions, and distances (interests and capabilities) for 14 different actors (Brazil, Burma, China, Cuba, Egypt, India, Indonesia, Israel, Jordan, Netherlands, Poland, United Kingdom, USSR, and United States). These actors were carefully selected to represent the different types and characteristics of all actors (Rummel, 1979: Section 3.1).
Now, each of these 197 equations define a situation in which behavioral dispositions and distance vectors are linked for a specific actor.6 The aim here is to determine whether general conclusions about situation, dispositions, and distance can be inferred. To do this requires some technique for aggregating these results.
The technique I applied can best be explained through reference to a subset of the equations. Table 9A.2 presents 21 canonical analyses (Rummel, 1977: 95-96, 148-149, 477-478; 1979; Choi, 1973). This organization and format were followed in constructing similar field-equation tables (not given here)7 for each of the other 13 actors.
First the results are organized on the left by theoretical components, which have been discussed in Chapters 4 and 7. Then, these theoretical components are divided into the empirical components which have been delineated in attribute or behavioral data.
Each of the canonical analyses employed empirical components from a factor analysis often done specifically for that canonical analysis. The empirical components thus defined often were similar, but differences also occurred across studies. Therefore, in aggregating the canonical results I had to be sure to keep similar input components together across the 197 canonical analyses. The organization of results by theoretical and empirical components helped in this. To explain this organization I will consider each theoretical component in turn.
For compulsory: this includes a "conflict behavior" component, in addition to nonmilitary components ("negative communication"; "negative sanctions"; "antiforeign behavior"; "deterrence"; "UN voting") and a military violence component.
In Table 9A.2, each numbered column defines a canonical equation (a situation) of the type shown in Figure 9A.1, and a rating for each component on it. These ratings measure two aspects of a canonical equation. One is the size of a canonical coefficient for a component; the other is the canonical correlation between the two canonical variates (the correlation between the sum of the left terms and the sums of the right terms, excluding residuals, shown in Equation 9A.4).
The ratings were formed in this way. Each canonical correlation and coefficient squared was transformed to integers as follows:

These integers then rate the dependent variance for a component behavioral disposition, or the variance of a component distance involved in a variate.10 Then, for each component on each variate a rating was calculated, where
rating = (integer for canonical correlation) x (integer for canonical coefficient).
This rating then measures the involvement of a component with the canonical variate, taking into account the canonical correlation. Thus, the better an equation fits behavioral dispositions, the more weight is given to the distances and dispositions involved.
These ratings for the twenty-one USSR equations are shown in Table 9A.2. Zeros are left blank; components omitted from an equation are indicated by an "X"; each rating is given the original sign of the associated canonical coefficient.
For example, the first, Equation 12, shows a high relationship between a power (power + size) distance of other states from the Soviet Union and its disposition toward compulsory behavior with them. Keeping in mind the interpretation of Figure 9A.1, then, the first equation shows that for the Soviet Union there is a situation in which
- Soviet perceptions give high weight to power distance, and
- Soviet expectations give high weight to compulsory dispositions.
The next problem is to aggregate these ratings in such a way as to provide an overall view of the results. This can be done in two ways.
First, one can count the frequency of occurrence of nonzero integers to the number that could occur because the appropriate components were included in the analyses. This ratio, labelled F in Table 9A.2, measures the probability that a distance or disposition will play some role in some situation. Thus, in Table 9A.2 the F columns show that power distance has the largest probability (12/2 1) of being situationally relevant for the USSR; contractual dispositions (for all four components = 11/21), the most likely disposition.
A second way of aggregating is to take into account the proportional size of the integers by summing their absolute values and dividing by the maximum possible. The result is the "P" columns in Table 9A.2. This measures the overall proportional salience of distances and dispositions to each other (because the ratings also involve the canonical correlation). Thus, for the USSR, power distance is proportionally (54/189) most salient; compulsory dispositions, proportionally the most salient disposition. Note that F and P thus can give different views of the same result, which is why they are both calculated.
Table 9A.2 is only a preliminary Table, however. It now enables me to compute the overall linkages for the USSR, as shown in Table 9A.3. This provides a tabulation of F and P for all possible cooccurring distances and dispositions, organized by components. The political and cultural components were combined in the third column, because both together reflect the meanings, values, and norms components of cultural space-time.
The entries in Table 9A.3 define for the USSR the percentage coincidence (F) of disposition and distance, or the cosalience (P) of both. Thus, distance in wealth and familistic behavioral dispositions could cooccur 21 times with a nonzero rating in Table 9A.2, but only cooccurred once. Therefore, (1/21) X 100 = 5% (rounded off), which is the figure shown in Table 9A.3. Similarly, to measure the cosalience the sum of the cooccurring distance and disposition rankings for an equation is calculated, summed for all equations, and divided by the maximum possible, which for wealth and familistic is (6 + 3)/378, which equals about 2%. For power and familistic disposition, this would be (2 + 2) + (6 + 6) + (6 + 2) + (3 + 9) divided by 378, or 36/378, which rounds off to 10%, as shown.
Thus, from all the entries in the Table, one can see that for the 21 equations power and political distances and contractual defense dispositions are most likely to go together (43% possible for each).
That is: in situations involving the USSR as an actor, the Soviets expect that the object's perceived power and political system-related interests and capabilities will lead to desirable outcomes for their more or less contractual-defensive behavior towards it.
Looking at the highest P however, the picture changes. For then power distance and compulsory disposition are shown to have the highest percentage salience (24% of possible). This means that: of all USSR expectations in situations involving her as actor, those jointly involving compulsory dispositions and her perception of another's power will be most salient.
On the margins of the Table are calculated the overall F and P for the distances and dispositions. Considering just the familistic component as an example, its F is the sum of all the cooccurrences in Table 9A.2 for the indicated distances G + 4 + 4 + 0 + 0 = 9) over the sum of the possible occurrences (21 + 21 + 21 + 17 + 10 = 90) in Table 9A.2. The overall F is thus 9/90, or 10% for familistic behavior, as shown on the margin in Table 9A.3. Similarly, the sum of the cosalience in Table 9A.2 for the familistic disposition (9 + 36 + 43 + 0 + 0 = 88) is divided by the sum of the maximum possible points (378 + 378 + 378 + 306 + 180) in Table 9A.2, which is 88/1620, or a rounded 5% as also shown on the margin.
From these marginals note that the USSR expectation of defense-related, contractual dispositions had the highest situational linkages to perceived distances; contractual-exchange the least. Moreover, looking at the bottom margin, perception of distance in power was the most predictive, or potent, distance vector in accounting for expected outcomes (dispositions); geographic distance the least.
The importance of Tables 9A.2 and 9A.3 here is in illustrating the process of synthesizing and aggregating the 197 canonical equations.11 I can now turn to the overall results.
9A.3 COOCCURRENCES AND COSALIENCE OF
SITUATIONAL DISTANCES AND DISPOSITIONS
Table 9A.4 presents the overall situational linkages for distances and dispositions. These are the marginal totals for behavior and distance and show which disposition is most situationally involved with distance vectors; which is least. Similarly, the totals indicate which perceived distance vectors are most and least potent for expectations. The values for the USSR are those given in Table 9A.3, and thus provide an initial point of comparison for understanding the calculation of all these numbers.
Again, these numbers are derived from the coefficients of 197 canonical equations, where the behavioral coefficients refer to expectations linking behavioral dispositions to situations in which an actor's perception gives weight to specific distances. Figure 9A.1 must be kept in mind. Thus, to observe that Table 9A.4 shows the contractual disposition is, for all actors, the most dependent on distance vectors means that in the diverse situations in which international actors behave, an actor's expectations give most weight to its contractual dispositions in terms of its situational perception of the distances (interest and capabilities) of the object state from it.
In describing the results here and in subsequent tables, I will usually leave implicit the reference to situational expectations and perceptions and describe the results in terms of dispositions-distance linkages. With this in mind, from Table 9A.4 a variety of conclusions can be drawn, a few of which are as follows.
- The contractual disposition is most often (F) and tightly (P) linked to distance vectors; military disposition the least.12
- Power is the most potent distance vector; geographic distance the least.
- For China and the United States, the familistic disposition is most often linked; for the USSR it is defense related, contractual dispositions.
- For the USSR, power is the most potent distance; for China it is the political; for the United States it is the political and cultural.
- For wealthy states, the contractual disposition is most often and tightly linked.
- For the poor, the wealth, power, and geographic distances are more potent, while political distance is more potent for the wealthy.
- For weak states the contractual disposition is more often and tightly linked to distance, and military disposition is linked less than for the powerful.
- For the weak, the wealth, power, demographic, and geographic distances are more potent, while political distance is more important to the powerful states.
- For liberal democratic as opposed to authoritarian (middle) and totalitarian states, the familistic disposition is most often linked, and the compulsory, nonmilitary disposition is the least linked; and power distance is least potent.
- For authoritarian states, the contractual disposition is most often linked, while wealth, demographic, and geographic distances are the most potent.
- For totalitarian states, the compulsory disposition is most often tightly linked, familistic the least; while wealth and demographic distances are the least potent.
Besides the overall results shown in Table 9A.4, of even greater interest is the cooccurrence and cosalience of specific distances and dispositions. These are shown in Tables 9A.5 and 9A.6. To help make rapid sense of all the figures, Table 9A.7 consolidates the more important results from these two tables, as well as from Table 9A.4.
A few of the many more important conclusions that might be drawn from these specific results are as follows.
- The power distance vector is most often and tightly linked to the compulsory disposition, especially for the nonpoor, powerful, or nonauthoritarian states.
- The political distance vector is most often and tightly linked to the contractual disposition, especially for China, middle wealthy, or liberal democratic states.
- The wealth distance vector is most often and tightly linked to the contractual disposition, especially for the USSR, poor, or middle powerful states.
- Of all linkages for the major powers and for the types of states, the power-contractual linkage most often situationally occurs for the poor; and the power-compulsory linkage is the tightest for the USSR.
However, the purpose here is not to distill propositions or conclusions from these tables. Rather, I only wish to present them along with the underlying logic so that others might, in terms of their own interests and at least for these actors and for this time period, use them to assess the relationship between an actor's situation expectations, behavioral dispositions, perceptions, and distance vectors (interests and capabilities); and to show that field theory and the field equations of behavior have an operational and empirical structure.
9A.4. THE MOST GENERAL EMPIRICAL EQUATIONS
OF SITUATIONAL DISTANCES AND DISPOSITIONS
The previous approach provides a specific and precise measurement of the linkages between distance vectors and behavioral dispositions. There is another way, however, of aggregating these equations, and that is in terms of the most general equations implicit in all these results. Given all the 197 equations, which best represent the overall, situational linkages?13
First, consider how a complete matrix of canonical equations would look for all significant equations and actors, as shown in Figure 9A.2. Each cell of the matrix would contain the canonical coefficient of the column attribute distance or disposition on the row-equation for a particular actor. For the analysis here this matrix was filled in with all canonical coefficients for 39 of the most significant equations across all 14 actors.
When we ask for the most general equations in the results, in effect, we mean to ask about what distances and behaviors most often cluster together in the matrix of Figure 9A.2. This is a question about the pattern of covariation in the Table, and can be answered using factor analysis: a way of determining systematically the most general equations for the canonical results is to factor analyze the matrix of canonical coefficients (the canonical structure matrix).14
The results of doing this are shown in Table 9A.8.15 Each of the four columns gives a general equation (situation) linking distances and dispositions implicit in the 39 significant equations (p <05, two-tailed) found for the 14 actors. Thus, for the first column we find
.86 (10) 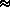 -.40(wealth) +.93 (totali.) + .96 (authori.)
+.48 (diversity) -.84(density) -.44(Catholic culture),
-.40(wealth) +.93 (totali.) + .96 (authori.)
+.48 (diversity) -.84(density) -.44(Catholic culture),
and this equation accounts for 22.5% of the variance in all the coefficients (measuring situational expectations and perception) among the 39 equations.
According to this equation, then: in general the wealthier, denser, more Catholic, less totalitarian, less authoritarian, and diverse the object state relative to the actor, the more an actor is disposed to join I0's with it.16 To turn this around, comemberships in international organizations tend to be a function of wealthy, densely populated, homogeneous Catholic, and democratic societies.
Or, in terms of the theoretical components and the higher coefficients: contractual dispositions are mainly a function of political and demographic distance vectors.
Finally, now bringing in the theoretical meaning of the coefficients and equation, this first equation says: there is a situation between states in which an actor expects that an object's perceived, relative political and density-related interests and capabilities will lead to desirable outcomes for the actor's contractual behavior towards it. This is the most general equation to be found in all the canonical linkage equations. And these are the three increasingly abstract ways (in terms of empirical components, theoretical components, or the expectations-disposition-perception-distance equation) in which it may be interpreted.
Turning now to the second most general equation in column four of Table 9A.8, which accounts for 15% of the variance, there is no need to lay out the equation here. We can simply interpret it as showing that: the weaker, smaller, stable, and more dependent on imports the object state relative to the actor, then the less an actor tends to transact with and export relatively to it.17 In terms of theoretical components: the less the power of the object states, the less an actor is disposed toward familistic and contractual behavior with it. And in terms of the theoretical meaning of the equation: there is a situation between states in which an actor expects that an object's perceived relative power-related interests and capabilities will lead to desirable outcomes for the actor's familistic and contractual behavior towards it.
The third most general equation accounting for 11.5% of the variance is shown in column 2 of Table 9A.8. This is purely a geographic distance linkage: the closer the actor is to the object states, the more disposed an actor to transact, align, and export relatively toward it. Or: the greater the familistic, contractual, and compulsory (nonviolent preparatory)18 disposition toward it. That is: an actor's perception of the geographic closeness of an object defines a situation in which it expects desirable outcomes of familistic, contractual, and compulsory behavior towards it.
The next general equation (column 3 of Table 9A.8) ties wealth and power to conflict. The poorer and weaker the object states relative to the actor, the less disposed the actor to direct negative communications, antiforeign behavior, and military violence towards it. And in terms of the theoretical meaning: there is a situation between states in which an actor expects that an object's perceived, relative wealth and power will lead to desirable outcomes for its compulsory behavior towards it.
The last general equation concerns aid: the poorer, more unstable, and non-Catholic the object state relative to the actor, the more aid the actor directs towards it. Or: some familistic behavior is a function of wealth, power (stability), and culture. And, finally: there is a situation between states in which an actor expects that an object's perceived, relative wealth, power, and cultural-related interests and capabilities will lead to desirable outcomes for the actor's familistic behavior towards it.
In summary and considering only the larger coefficients of the distances in Table 9A.8 and the empirical components, we find general situations (equations) separately linking dyadic conflict (excluding alignment) and cooperation. For conflict, we find a conflict-wealth-power situation where dyadic conflict is purely related to the wealth and power distances.
For cooperation, we find four different general situations. The most general is an LO.-politics-density situation, where the tendency toward comemberships in international organizations is largely a function of the democratic political characteristics of the object and its relative density. Second, we have a transactions-alignment-exports-geographic distance situation whereby closer states are disposed toward more cooperative interaction. Third, there is a general transaction-export-power-size situation, relating dispositions toward dyadic transactions and relative exports mainly to power and size. Finally, the weakest linkage is an aid-wealth-stability-Catholic situation.
These five general situations underlie the 39 significant equations (situations) for the 14 actors, 1950-1965. These show that the data contain patterned relationships between distance vectors and important international, empirical, behavioral dispositions. Wealth, power and politics indeed relate to transactions, conflict, trade and alignment--to familistic, contractual, and compulsory dispositions.19 Clues to some of these relationships are suggested in Tables Table 9A.4 to 9A.8.
Finally, there is one more aspect of Table 9A.8 to note: the communalities (h2). The larger the commonalities, the more involved a distance vector in the general situations (equations) or the more a behavioral disposition is weighted by situational expectations. Thus, we find that the distance most involved in these general situations is authoritarianism (.95), next is power (.91), and totalitarianism (.88) is third. By far, expectations most highly weight dispositions to transact (.94), export (.93), and to cojoin international organizations (.90). The worst distance (in finding general, across actor situations) is geographic; the worst disposition is aid.
Considering dyadic international behavioral dispositions as a whole, then, the most productive distances to focus on are political and power. Indeed, these are the elements students of international relations have traditionally used to explain international behavior. And those behavioral dispositions most tied to these distances are transactions, exports and international organization comemberships.
9A.5. THE MOST COMMONLY SHARED EMPIRICAL
EQUATIONS AMONG GROUPS OF ACTORS
The previous Section has shown what general situations exist among the 39 significant dyadic situations for the 14 actors. This Section will focus on the similarity between actors in the situations they manifest. Are some situations similar for China and the USSR; what about the USSR and the United States? Is there a similarity in situations between developed and underdeveloped, nontotalitarian and totalitarian, or powerful and weak actors? The way to determine this is to Q-factor analyze the same complete matrix of canonical coefficients exemplified in Figure 9A.2 and used to generate Table 9A.8. We factor by rows (the actors and equations) rather than by columns (the distance vectors and behavioral dispositions), and the resulting dimensions (when rotated) define the clusters of actors with similar equations (situations).
These results are shown in the upper half of Table 9A.9, where the coefficients are the correlations between the actors and each of the common groups (columns). The bottom half of the Table presents the general equations (factor scores) manifesting the situational similarity of actors within each group.
There are seven groups of actors, the first being by far the largest. It includes all of the 14 actors except the Netherlands, USSR and United States; and Brazil and Indonesia are central members. Regarding the equation for this group, the signs of the factor scores can be interpreted the same as the coefficients of Table 9A.8.20 We, therefore, can read the general equation for those actors in this group and the empirical components as: the less the object's power, and the greater its diversity and Catholic culture relative to these actors, the more these actors tend to join international organizations with, and the less they are disposed to transact with and relatively export to, the object. This is a group of actors which tend to focus their cooperation on international organizations to the exclusion of exports and transactions if the object state is weak, diverse and Catholic relative to them. Power here is the main distance vector.
The theoretical meaning of this equation is this: there is a situation between states in which Brazil and Indonesia, especially, but also Israel, India, United Kingdom, Jordan, Egypt, Cuba, Burma, China, and Poland expect that an object's perceived, relative power, Catholic culture, and diversity-related interests and capabilities will lead to desirable outcomes for their comembership in international organization, and undesirable outcomes for their transactions with and relative exports to the object.
The second group (column 2 in Table 9A.9) also includes most states, but now China, Egypt, Jordan and the USSR are omitted. United Kingdom, Netherlands, Brazil, Israel and the United States are central members, making this appear a dominantly Western group. This group cooperates with other states mainly in line with their wealth. Specifically, the wealthier the object state relative to them and the less authoritarian and the denser it is, the greater their transactions and relative exports to it and the more they cojoin international organizations with it. Theoretically: there is a situation between states in which the United Kingdom, Netherlands, Brazil, Israel, and the United States particularly, but also Indonesia, India, Cuba, Burma, and Poland expect that an object's perceived, relative wealth, nonauthoritarianism, and density-related interests and capabilities will lead to desirable outcomes for their transactions and international organizations comemberships with, and relative exports to, it. This collection of actors can be called a cooperation-wealth situational group.
The third group (column 3 in Table 9A.9) involves Egypt, Israel, Jordan and the Netherlands, and appears mainly a Middle East group. To these actors the relative totalitarianism of the object states is crucial in their alignments. That is, the less totalitarian and import dependent the object state and the further away, then the more they are inclined to align with it. Leaving out the theoretical interpretation, this may simply be labeled an alignment-politics situational group.
The remaining groups involve two actors. There is one for China and the USSR, showing that they both transact and align with, export more to, and send lesser negative communications to relatively poor, weak, totalitarian and authoritarian states. They form a cooperation-wealth-politics-power situational group. Another group comprises Egypt and Jordan, who align and have conflict more with object states, the more relatively import dependent, non-Catholic and closer geographically they are. Egypt and Jordan constitute a cooperationconflict-geographic distance situational group. Finally, the two groups involving only the United Kingdom and the United States show that they have two different linkage equations, or situations, in common. One shows that they have more military violence with the larger, relatively more diverse and denser object states; the other shows that they direct less negative communications to and join fewer international organizations with object states, the less totalitarian, authoritarian, diverse and Catholic they are and the more unstable and import dependent. The United Kingdom and United States form conflict-stability-diversity situational groups.
In summary, we find that certain actors share specific situations in which they are similarly affected by distances. The same distances are linked to the same dyadic behaviors in the same ways. There are seven such groups of actors, some showing common situations involving organization memberships and relative power; involving cooperation and relative wealth; involving alignment and relative politics; involving cooperation and relative wealth, politics and power; involving cooperation and conflict and geographic distance; and involving conflict, stability and diversity.
NOTES
* Scanned from Appendix 9A in R.J. Rummel, Understanding Conflict and War: Vol. 4: War, Power, Peace. For full reference to the book and the list of its contents in hypertext, click note [13]. Typographical errors have been corrected, clarifications added, and style updated.
1. For the mathematical development of the canonical model in relation to field theory, see Rummel (1977: Section 4.4).
2. Technically, I am assuming that the number of canonical variates (situations) will equal the number of input components in the dependent (behavioral dispositions) matrix. This is a necessary assumption of the theory, where a certain measure of free will and specific influences are involved in each situation. Thus, theoretically, the trace correlation should never be 1.00: all the common variance in behavioral dispositions should never be completely dependent on distance vectors, and the number of variates will equal the number of behavioral components. A second auxiliary assumption is that the number of input behavioral components is less than or equal to the number of distance vectors. This is a theoretical assumption: common behavioral space-time is a subspace of the common international (attribute) space-time and therefore its dimensionality cannot exceed that of attributes, i.e., the number of distance vectors.
3. Both my ontology and epistemology are inseparably linked through this approach, which was philosophically developed in Vol. 1: The Dynamic Psychological Field. That is, expectations and perceptions are aspects of the dynamic psychological field. They can be only indirectly known by observers from their manifestations--their traces on the plane of phenomena. Here, the manifestations are the linkages between behavioral disposition and distance vector, i.e., the canonical coefficients. As to why this particular linkage should display expectations and perceptions was the burden of Vol. 1: The Dynamic Psychological Field and Vol. 2: The Conflict Helix to establish. For example, see Part III of Vol. 1: The Dynamic Psychological Field.
4. Reported here for the first time are part of the results of Project 45, described in Appendix I.
5. Many more canonical analyses than these have been done, but not all are distinct enough to include here. For example, some simply involved reanalyzing the same data with minor changes in methodology.
6. A number of criteria entered into selecting each equation from those generated in the analyses: its canonical correlation, significance test, meaningfulness, and the size of its canonical coefficients.
7. Note omitted.
8. The names in parenthesis here and below are those given by the scientist to the relevant component in his analysis.
9. In previous works and in Chapter 4 I kept the primary power component separate from other components, such as domestic conflict and trade dependence, which have a secondary relationship to a state's coercive power. Here, however, empirical components are classified under the most relevant theoretical label for the purpose of linking them to behavioral dispositions, and the secondary nature of the empirical component is then crucial.
10. Generally, structural canonical coefficients were used. These are correlations between component and variate, and thus their square measures their variance in common.
11. There is a difference between some of the F and P values shown for the USSR in Table 9A.3 (and also for the U.S. in subsequent Tables 9A.4 to 9A.7) and those values in Tables 5 and 7 of my "Soviet Strategy and Northeast Asia," Korea and World Affairs: a Quarterly Review, Research Center for Peace and Unification, Seoul, Korea (Vol. 2, Spring, 1978). Where differences occur, those in the article are in error (i.e., for USSR demographic weights and U.S. wealth and geographic distance weights in Table 5; and for all except U.S. cooperative weights in Table 7). The corrected figures (weights) do not in any way alter the points made in the article, and if anything slightly strengthen the conclusions.
12. The reason for this low linkage of military action to distance vectors is that it is a phase in conflict behavior. Distances affect the initiation, and to a certain extent, the intensity of conflict; but whether a conflict will escalate to violence is related to a number of contextual, nondistance-vector, factors. See Appendices 15A and 16B, particularly Propositions 15.3 ; 16.9 to 16.11; and 16.16 to 16.22 .
In general, cooperation (familistic and contractual behavior) has a better linkage to distances (Table 9A.4) or attributes (Rummel, 1972; Chapter 13) than conflict (compulsory behavior). See also Vasquez (1975: Table 12). The reason for this is that cooperation is a particular fit to attributes (interests and capabilities), whereas, conflict is the universal means by which this matching takes place. The difference is that between structure and process.
13. The following is a revision of Rummel (1979: 9).
14. In the analysis, the correlations between variables and canonical variates were used (the canonical structure matrix) rather than coefficients.
15. I am indebted to Peter Sybinsky for originating all the analyses reported in this Section and the next.
16. The distance vectors can be interpreted easily by reference to the object nation if the sign of the distance coefficient is reversed (see Rummel, 1979: Chapter 8). Thus, contractual dispositioni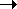 j
j
= +.63 (wealth distancei-j), can be interpreted as: the less wealth of j, the more the disposition of i to contract with j. If the sign of .63 were originally negative, then the equations would read: the more wealth of j, the more the disposition of i to contract with j.
17. The relationship between import dependency and dyadic, relative exports is not tautological. A State may have relatively low imports in relation to its total trade (thus being trade-independent), while another is sending to it high exports relative to that state's GNP. Indeed, were there any tautological relationship, this correlation should be the opposite of that shown.
18. Alignment is a preparatory phase of conflict behavior. See Chapter 15.
19. This is a revision of Rummel (1979: Chapter 10).
20. See Note 16.
For citations see the Vol. 4: War, Power, Peace REFERENCES
Go to Home Page
j