TOWARD A NEW ELECTROMAGNETICS PART III

-- CHARGED-MASS-SYSTEM VECTOR --
-- (Figure 4) --

The third type of vector we meet is the vector mass system where the mass is charged. First, we point out a serious error in present electromagnetic (EM) theory. That is, in present theory it is implicitly assumed that

q ≡ q_m

(8)

In other words, "charge" and "charged mass" are erroneously assumed to be identically the same thing.
In the days when electricians were playing with pith balls and striving to uncover the secrets of electricity, they knew nothing at all about the virtual state, and consequently nothing about a "virtual particle flux" on a particle of mass causing (and comprising) the "charge" of that mass.
Today, of course, we know from particle physics and quantum mechanics that the "charge" on an observable particle of mass IS due to a flux of virtual (nonobservable) particles on and off the mass of the observable particle (see figure 5). A charged mass is thus presently known to be a SYSTEM: a massless charge flux, coupled to a bare particle (chargeless mass) constitutes a "charged particle."

Figure 5. The "charge" on an electron mass consists of
a flux of virtual particles on and off the mass.

Thus, actually the "charge" is the virtual (unobservable, or SPATIO-TEMPORAL) flux to and from the observable SPATIAL particle of mass. So, rigorously,

q q_m

(9)

But instead,

q ≡ [d/dm(q_m)]

(10)

and this is a definition and therefore an identity. This definition alone affects all present electromagnetics theory.
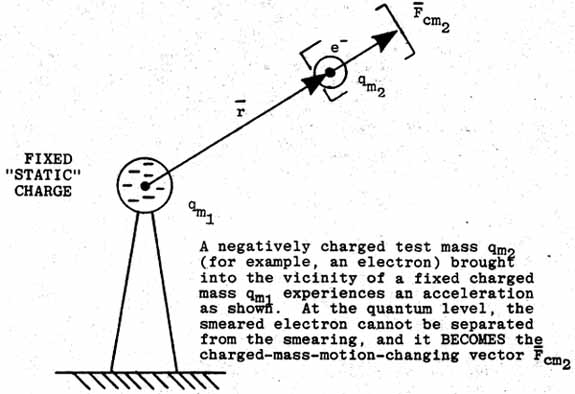
To illustrate: In founding electrical theory, early scientists dealt with forces generated by charged masses (for example, charged pith balls). They later extrapolated the experimental results they obtained (or thought they obtained) with the smallest charged mass, a charged particle. In Figure 6, I show the classic situation for derivation of the idea of E-field (except we have used an electron for our test charge, rather than a pith ball).

Figure 6. A test charge (charged mass) brought near a fixed charge
(charged mass) experiences an acceleration.

Now note that what actually happens is that the unrestrained test charge becomes a CHARGED MASS SYSTEM VECTOR (a "smeared charged mass-motion changing"). The "test charge" BECOMES a charged mass force vector; it does not have a separate geometer's vector "appear on it." What actually happens is shown in Figure 7.

Figure 7. A charged-mass-system vector.

That is, in the simplest (nonrelativistic) case, for an electron what happens is

(11)

and this is a DEFINITION. That is, considered instantly, the electron exists as a charged-mass electrical force CONSISTING OF/COMPRISED OF a charge flux q_e canonically coupled to a mass, with that subsystem then canonically coupled to a spatial acceleration vector, ALL AS A SINGLE ENTITY, WITHOUT ANY "SEAMS" BETWEEN ITS "PARTS." The _cm IS THE ELECTRON SYSTEM ITSELF; it is NOT a "spatial vector." Rigorously, it does not exist in the absence of the smeared electron mass, a priori.
Again, in assuming this force exists in the absence of the smeared mass of the moving particle, electromagnetics theory is in serious logical error.
Referring back to Figure 6, we see that, if we repeat the experiment many times and with the test charge in many locations, we have the situation shown in Figure 8.

Figure 8. Repeating the "test charge" experiment.

It is found that, rigorously,

(12)

where _cm is a charged mass system vector. Erroneously, this has been stated one way or another as

(13)

where is assumed to be a spatial system vector. Further, this confusion has been carried over into the definition of the -field as:

(14)

In this definition, -- which is a charged mass system vector -- has been confused as a charged spatial system vector, where is regarded simply a spatial system vector! Actually, the definition of the -field should be

(15)

where _cm is a charged mass system vector. Failure to properly define the -field has caused the conception of the -field to be falsely perpetuated as existing in vacuum.
           The -field is TREATED this way in present EM theory. Hence present theory falsely assumes that the observable -field can exist in vacuum.
          What actually exists in space, -field-wise, is a special kind of ordered virtual state pattern in a series of spinning "scalar" fields. This virtual state pattern or "shadow vector" field will be explained later.
          Note again that one cannot have a "force vector" existing in vacuum - a priori.
          However, assume for a moment that one could have a massless force vector, as presently assumed. Let this force vector appear at a point in the vacuum. Since the vacuum has zero observable mass, it would have zero inertial resistance to this hypothetical observable force hence the observable force would instantly produce an "infinite" acceleration of its point of application, vanishing with it into the distance. Therefore our fictitious force would disappear the instant it appeared! In any case, it could not be retained at a point in the vacuum for any finite length of time, however small.
          The direct implications are that (1) something other than an observable electrical force field exists in the vacuum, and (2) there must exist a more fundamental mechanism by which this "something else" generates or CREATES a change on/of an accelerating electrically charged mass particle. (Note again that at the basic level, any particle of mass is ALWAYS quivering and accelerating, from quantal fluctuation considerations alone.) Causality has no arrow microscopically.²³

Next Page